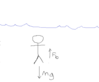
This question also gave me a headache, especially since it's easy to overthink (several of the practice test questions were!). I got really hung up on whether the person was fully submerged but not sunk, which would influence the way you think of the free body diagram. Approaching it conceptually ended up being the easiest. An object that is submerged (assuming it's submerged, not sunk) will have its apparent weight in air (Fg of the object) equal to the weight of the fluid that it displaces (in other words, the buoyant force FB). A free body diagram for this would be two perfectly balanced forces, with the buoyant force pointing up and force of the object pointing down.
So mathematically, let's write that as FB = Fg. We can go a step further and break that down into FB = (mfluid displaced)(g) and likewise, Fg=(mobj)(g). Now how can we relate this to the densities of the fluid and the object? Let's say p= density because I don't have a rho key and also I'm going to stop typing the whole fluid displaced thing and just write "fluid."
pobj= mobj/Vobj
pfluid = mfluid/Vfluid
But let's solve these for the masses, since we want to relate the forces to the densities and this way we can substitute densities into the equations for the forces seen in the paragraph above. So:
mobj = pobj(Vobj)
mfluid = pfluid(Vfluid)
Now substitute into the equations for the forces and we get:
Fg = pobj (Vobj) (g)
Fb = pfluid (Vfluid) (g)
Okay! Now we have something relating forces to densities. The question asks what is the density of a human body proportional to, so let's set up a proportion using these equations, relating forces to densities.
Fg/Fb = (pobj) (Vobj) (g) / (pfluid) (Vfluid) (g)
which I'm going to write for clarity's sake as:
(pobj) (Vobj) (g) / (pfluid) (Vfluid) (g) = Fg/Fb
We can simplify this further. The (g) cancels and so do the volumes--that's because a fully submerged object MUST displace a volume of fluid that's equal to its own volume--conservation of space if you will 🙂 Therefore the volume of the object is equal to the volume of the fluid displaced. Now we've got a nice simple equation:
pobj / pfluid = Fg /Fb
This is actually a really useful equation to memorize, because it follows intuition (for example, let's say the density of the object is greater than the fluid, then the weight of the object is greater than the buoyant force and so the object will sink...etc). How does this relate to the problem? Well, the density of the fluid here is 1 because the fluid is water. Fg is the weight of the object (the person) in air and Fb is the buoyant force, which someone above has pointed out is Wair-Wwater. Plug it all in and you get the answer: density of the object = Wair/Wair-Wwater
Hope that helps!