medium yus
New Member
- Joined
- Jun 1, 2018
- Messages
- 4
- Reaction score
- 0
I recently checked out a document from UCLA a which shows the pKa's of each amino acid. I know that each protein's environment can have a affect on pKa's, or so I was told, but that is besides the point.
My question is in regards to figuring out charge, specifically for tyrosine.
Assumptions:
1. Phenol = 10 pKa
2. Amine = 9 pKa
3. Carboxylic Acid = 4 pKa
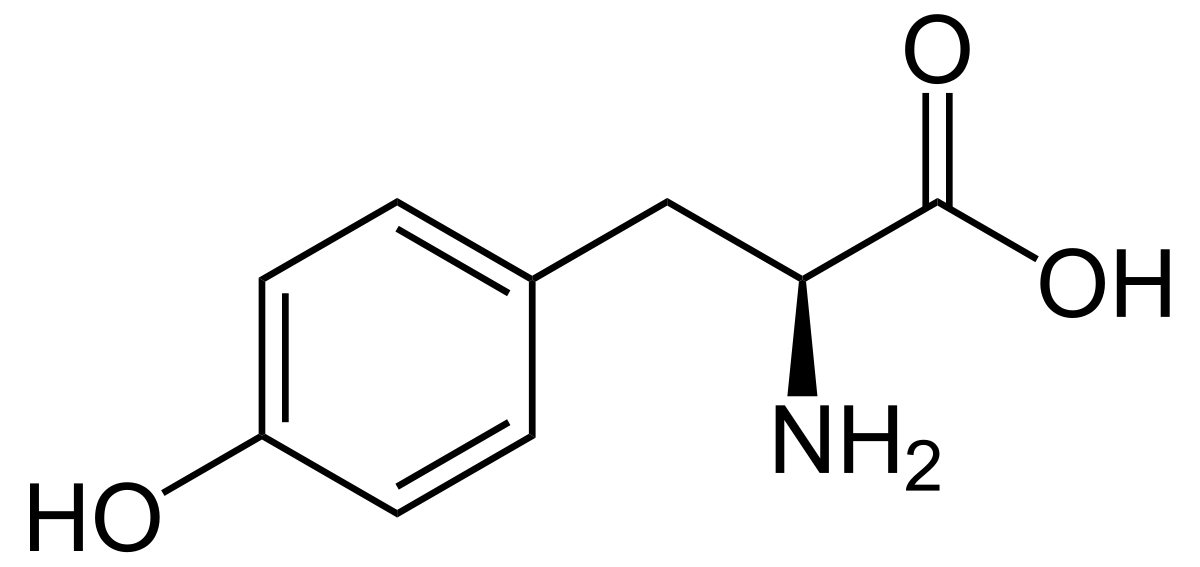
The above compound is neutral, but I am assuming this is under strongly acidic conditions. My assumption is that its isoelectric point is 5.64 because the amine is protonated and the carboxylic acid is deprotonated while the phenol is still protonated. (Right? See my thought process below)
As pH increases to physiological pH the amine should lose its hydrogen only slightly (the phenol should lose it very, very slightly as well) while the carboxylic acid remains at a negative charge.
Once we reach a pH of say 10, which I arbitrarily chose, tyrosine should be considered negative.
My question is in regards to figuring out charge, specifically for tyrosine.
Assumptions:
1. Phenol = 10 pKa
2. Amine = 9 pKa
3. Carboxylic Acid = 4 pKa

The above compound is neutral, but I am assuming this is under strongly acidic conditions. My assumption is that its isoelectric point is 5.64 because the amine is protonated and the carboxylic acid is deprotonated while the phenol is still protonated. (Right? See my thought process below)
Phenol: (a) = 1 / ((10^(10−5.64)) + 1) = .004 % deprotonated (.004 * -1 = -.004)
Carboxylic Acid: (a) = 1 / ((10^(4−5.64)) + 1) = 97.7% deprotonated ( -.977)
Amine: (a) = 1 / ((10^(9−5.64)) +1) = .04 % deprotonated (.96 * +1 = ~+.96)
Total Charge Estimate = -0.021
Carboxylic Acid: (a) = 1 / ((10^(4−5.64)) + 1) = 97.7% deprotonated ( -.977)
Amine: (a) = 1 / ((10^(9−5.64)) +1) = .04 % deprotonated (.96 * +1 = ~+.96)
Total Charge Estimate = -0.021
As pH increases to physiological pH the amine should lose its hydrogen only slightly (the phenol should lose it very, very slightly as well) while the carboxylic acid remains at a negative charge.
Phenol: (a) = 1 / ((10^(10−7.4)) + 1) = .25 % deprotonated (.25 * -1 = -.25)
Carboxylic Acid: (a) = 1 / ((10^(4−7.4)) + 1) = 99.96% deprotonated (~ -.9996)
Amine: (a) = 1 / ((10^(9−7.4)) +1) = 2.45 % deprotonated (.9755 * +1 = +.9755)
Total Charge Estimate = -0.2741
Carboxylic Acid: (a) = 1 / ((10^(4−7.4)) + 1) = 99.96% deprotonated (~ -.9996)
Amine: (a) = 1 / ((10^(9−7.4)) +1) = 2.45 % deprotonated (.9755 * +1 = +.9755)
Total Charge Estimate = -0.2741
Once we reach a pH of say 10, which I arbitrarily chose, tyrosine should be considered negative.
Phenol: (a) = 1 / ((10^(10−10)) + 1) = 50% deprotonated (.5 * -1 = -.5)
Carboxylic Acid: (a) = 1 / ((10^(4−10)) + 1) = 99.99% deprotonated (~ -.9999)
Amine: (a) = 1 / ((10^(9−10)) +1) = .90% deprotonated (.1 * +1 = +.10)
Total Charge Estimate = -1.3999
So here is my question--assuming everything above is correct. Do you think we should only be expected to know an amino acid's charge at physiological pH and if it is not quite negative or neutral, such as in the case of tyrosine, that we treat it as neutral?Carboxylic Acid: (a) = 1 / ((10^(4−10)) + 1) = 99.99% deprotonated (~ -.9999)
Amine: (a) = 1 / ((10^(9−10)) +1) = .90% deprotonated (.1 * +1 = +.10)
Total Charge Estimate = -1.3999
