- Joined
- May 25, 2018
- Messages
- 131
- Reaction score
- 153
#278 from EK 1001 Physics.
In this one, the magnitude of angles are unknown, and are assuming the system is in equilibrium.
Therefore, looking at horizontal aspects of T1 and T2 you can set T1cos(theta) = T2cos(theta). Cancel out cos(theta) to get T1=T2.
Am I missing something here? I don't understand where in the world they pulled out T1>T2
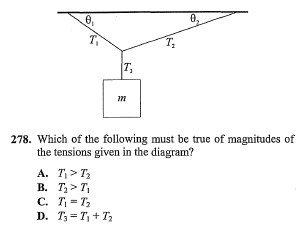

In this one, the magnitude of angles are unknown, and are assuming the system is in equilibrium.
Therefore, looking at horizontal aspects of T1 and T2 you can set T1cos(theta) = T2cos(theta). Cancel out cos(theta) to get T1=T2.
Am I missing something here? I don't understand where in the world they pulled out T1>T2
