This question has to do with the Arrhenius equation (ignoring the "A"), k = e^ -Ea/RT. This equation is frequently provided on standardized exams.
Two reactions a --> products and b --> products.
We will assume identical concentrations for both a and b. The rate laws for each are: Rate = k[a](raised to the x) and Rate = k (raised to the y which may or may not be the same as x).
According to the Arrhenius equation the rate constant, k, is related to Ea and temperature. Since the temperatures are stated to be the same for the two reactions, if the Eas are different, the rate constants, k, must be different since R is a constant.
So how can the rates be the same if the rate constants are different? The order of the reactants must be different for the two reactions i.e. first order for "a" (x = 1) and second order for "b" (x = 2, or something like that). But this isn't part of the answer, just an explanation for the original premise.
From the Arrhenius equation, the larger the Ea, the smaller will be the k. This is a math thing based on the form of the equation. So the reaction with the larger Ea will have a smaller rate constant, k, and be slower (in general unless other things like order or concentration are introduced as changes).
The important thing is how the rate constant changes with temperature. This may be more conceptually obvious to someone that is mathematically inclined, but you can make up some numbers and check it out empirically. It turns out that a reaction with a larger Ea, when you change the temperature, will have a larger effect on the rate constant, k. Recall that the rate constant changes with temperature.
That is, the reaction with the larger Ea will have a bigger change in the rate constant (making it faster) than the reaction with the smaller Ea (which will also be faster, but not as much).
Using the Arrhenius equation, you can see this by using three values of temperature (273, 283 and 373) and two values for Ea (100 J and 200 J). Then solve for k and plot lnk versus 1/T according most general chemistry textbooks. The slope is the (negative) value for the energy of activation (divided by R, a constant) or -Ea/R. For the reaction with an Ea of 100J, a slope of -11.91 is obtained. For the reaction with an Ea of 200J, a slope of -24.16 is obtained. Bigger slope equals bigger change (effect).
Clearly this problem wasn't meant to be solved by plotting values. So I think it is the ability to see how the value of k is affected by a change in the denominator (temperature) of the exponent in the Arrhenius equation that, again, for someone mathematically versed, could be seen conceptually without using numbers and plotting them. Or simplistically a bigger energy of activation has a bigger slope (with the variables ln k and T) and thus will change more with temperature.

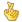 - i hope!
- i hope!