- Joined
- Apr 23, 2004
- Messages
- 646
- Reaction score
- 4
This thread is a reference work, not a discussion. It comprises answers to Frequently Asked Questions (FAQs) about the physics portion of the MCAT, and write-ups of topics that give many students trouble on the test. Some of the posts are detailed, because the topics are non-trivial. Those in-depth posts are intended to guide your studying, not to substitute for other materials. We hope that this thread becomes a permanent resource for the SDN community.
It will be some time before this thread is completed. If you want to suggest a topic for inclusion (which request I will happily consider), please PM me. Meanwhile, readers and contributors should expect stuff to move around on a daily basis while construction continues.
Please do not post in this thread without permission. If you have permission, fire away, but be willing to have your posts edited, for format and content.
Thread moderated by: Shrike. Shrike is a full-time instructor for The Princeton Review; he has taken the MCAT twice for no good reason, scoring 14 on the physical sciences section each time. He majored in mathematics and economics, minored in physics, and spent several years accumulating unused school experience (in law).
**********
Table of Contents
It will be some time before this thread is completed. If you want to suggest a topic for inclusion (which request I will happily consider), please PM me. Meanwhile, readers and contributors should expect stuff to move around on a daily basis while construction continues.
Please do not post in this thread without permission. If you have permission, fire away, but be willing to have your posts edited, for format and content.
Thread moderated by: Shrike. Shrike is a full-time instructor for The Princeton Review; he has taken the MCAT twice for no good reason, scoring 14 on the physical sciences section each time. He majored in mathematics and economics, minored in physics, and spent several years accumulating unused school experience (in law).
**********
Table of Contents
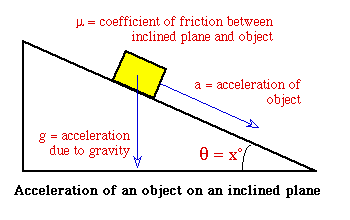
- Post 23: Acceleration, Speed, and Velocity
- Post 13: Applications of Thermodynamics (First Law of Thermodynamics, Calorimetry and Refrigerators--from Gen Chem Explanations Thread)
- Post 16: Buoyancy: Solving Problems
- Post 27: Capacitors
- Post 28: Capacitors: Examples
- Post 14: Centripetal and Centrifugal Forces
- Post 04: Conservation Laws
- Post 17: Conversion Units and Dimensional Analysis
- Post 19: Conversion Units II
- Post 22: Coulomb's Law
- Post 25: Electric Fields
- Post 26: Electric Potential and Work (Electrostatics)
- Post 09: How to Read Physical Science Passages
- Post 20: Neurotransmission from a Physics Perspective
- Post 10: Newton's Third Law
- Post 03: Optics Problems: How to Solve Them
- Post 21: Pendulums and Springs: Tension
- Post 15: Physics Constants Necessary for the MCAT and OAT
- Post 11: Physics Equations
- Post 12: Physics Equations II
- Post 13: Physics Equations III
- Post 08: Right Hand Rule for Magnetic Fields
- Post 18: Simple Machines
- Post 24: Solving Slope Problems
- Post 02: Solving Trig Functions without a Calculator
- Post 07: Tension: Ropes, Cables, Wires, Strings
- Post 05: Waves: Transverse and Longitudinal
- Post 06: Weightlessness


 .
.