LOL, I remember thinking "what the fork" when I first saw this entire passage.
This is exactly the type of reasoning you need to master for the MCAT, so I recommend considering both a conceptual answer and a numbers approach.
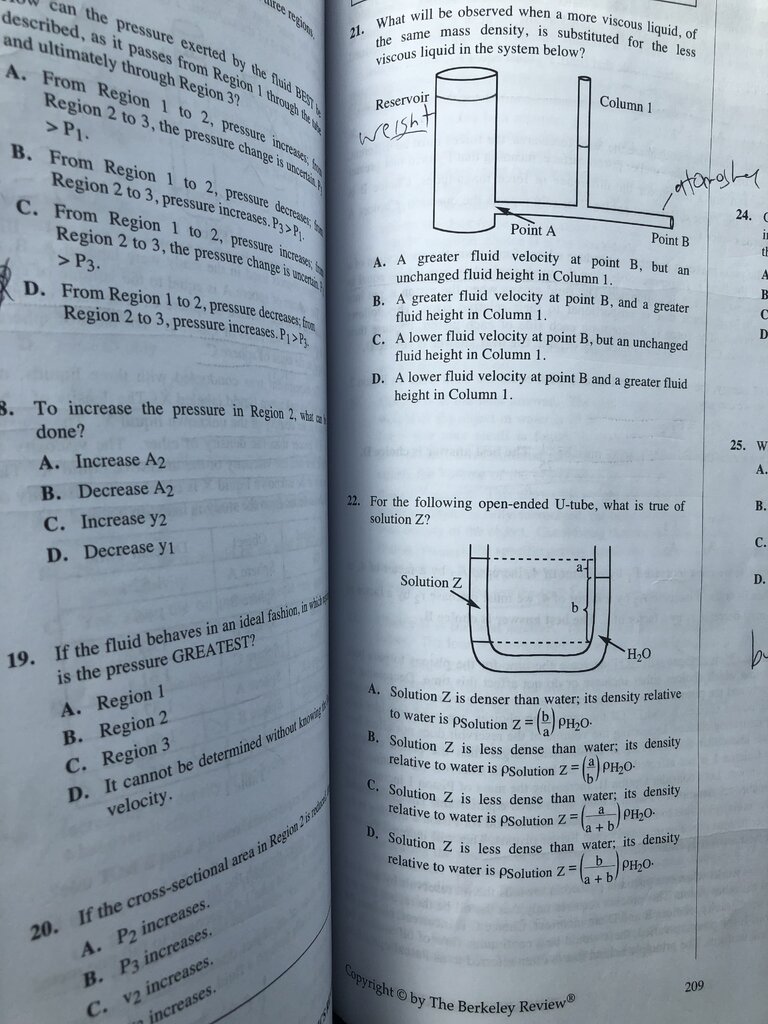
Conceptually speaking, because the fluid is not moving, the pressure of the column of solution Z on the left (with a height of a+b) must equal the pressure of water above the same baseline (giving it a height of b.) So picture in your mind Z with height (a+b) equal to water with height b.
The pressure of a column of fluid is based on rho x g x h and we know that g is a constant, so on the Z side there must be a slightly lower density (because it has a slightly higher height) than on the water side (where there is a slightly lower height.) The relative heights are equal to the relative densities, so given that a is a small number compared to b, we can see that (a+b) : b is only slightly greater than 1. The ratio of the densities of water and Z must also be that same small value of (a+b) : b, not b : a (way too big) or (a+b) : a (even bigger.)
I think their math solution is pretty good, but I personally like thinking about it conceptually.
Bring your 2026 application questions to our open office hours with Emil Chuck, PhD, Director of Advising Services for HPSA, and get them answered live. Personal statements, secondaries, interview prep, school list strategy: all fair game. Sunday, May 17 at 9 p.m. Eastern.
